Mass Balancing (Two-Product Formula)
Jump to navigation
Jump to search
Description
This article describes the classical two-product formula for estimating the separation efficiency of a process.
Model theory
Excel
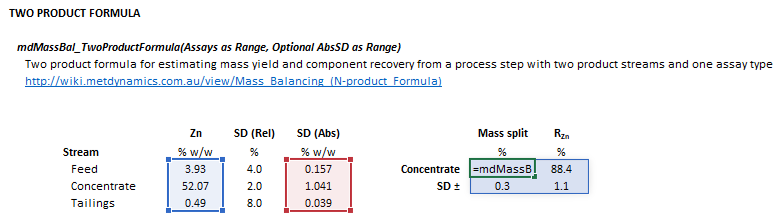
The two-product formula may be invoked from the Excel formula bar with the following function call:
=mdMassBal_TwoProductFormula(Assay as Range, Optional AbsSD as Range)
Invoking the function with no arguments will print Help text associated with the model, including a link to this page.
The input parameters and calculation results are defined below in matrix notation, along with an example image showing the selection of the same cells and arrays in the Excel interface:
|
| ||||