|
md>Scott.Munro |
| Line 9: |
Line 9: |
| [[File:PartitionWhitenWhite1.png|thumb|450px|Figure 1. Screen partitions to oversize, with <math>D_1=50\text{ mm}</math>, <math>D_2=20\text{ mm}</math>, <math>R_{\rm f}=0.2</math> and <math>\lambda=0.2</math>. Note how the particle aspect ratio, <math>d_{\rm AR}</math>, shifts the partition curve, allowing irregular particles to pass slotted apertures that would otherwise be retained.]] | | [[File:PartitionWhitenWhite1.png|thumb|450px|Figure 1. Screen partitions to oversize, with <math>D_1=50\text{ mm}</math>, <math>D_2=20\text{ mm}</math>, <math>R_{\rm f}=0.2</math> and <math>\lambda=0.2</math>. Note how the particle aspect ratio, <math>d_{\rm AR}</math>, shifts the partition curve, allowing irregular particles to pass slotted apertures that would otherwise be retained.]] |
|
| |
|
| The Whiten and Whit expression for recovery to screen oversize is: | | The Whiten and White expression for recovery to screen oversize is: |
|
| |
|
| :<math>
| | {{Model theory (Text, Whiten and White Partition Curve)}} |
| E_{{\rm oc}i} =
| |
| \begin{cases}
| |
| \exp (- n_L L f_{\rm o} \dfrac {\big [D_1 - \bar d_i \big ] \big [D_2 - \bar d_i \big ]}{D_1 D_2}) & \bar d_i < D_2\\
| |
| 1.0 & \bar d_i \geq D_2\\
| |
| \end{cases}
| |
| </math>
| |
| | |
| where:
| |
| * <math>i</math> is the index of the size interval, <math>i = \{1,2,\dots,n\}</math>, <math>n</math> is the number of size intervals
| |
| * <math>E_{{\rm oc}i}</math> is the corrected fraction of particles of size interval <math>i</math> in the feed reporting to the oversize stream (frac)
| |
| * <math>\bar d_{i}</math> is the [[Conversions|geometric mean size]] of the size interval <math>i</math> (mm)
| |
| * <math>n_{\rm L}</math> is the number of trials per unit length parameter (/m)
| |
| * <math>L</math> is the length of the screening area (or panel) in the direction of flow (m)
| |
| * <math>f_{\rm o}</math> is the fraction open area of the screen deck/panels (m<sup>2</sup>/m<sup>2</sup>)
| |
| * <math>D_1</math> is length (the longer side) of the screen deck/panel aperture (m)
| |
| * <math>D_2</math> is width (the shorter side) of the screen deck/panel aperture (m)
| |
| | |
| Whiten and White's equation was modified by Dehghani et al. (2002) to include a term for irregularly shaped particles, and is generalised to:{{Dehghani et al. (2002)}}
| |
| | |
| :<math>
| |
| E_{{\rm oc}i} =
| |
| \begin{cases}
| |
| \exp (- n_L L f_{\rm o} \dfrac {\big [D_1 - \sqrt{2} \bar d_i \cos \theta \big ] \big [D_2 - \sqrt{2} \bar d_i \sin \theta \big ]}{D_1 D_2}) & \sqrt{2} \bar d_i \sin \theta < D_2\\
| |
| 1.0 & \sqrt{2} \bar d_i \sin \theta \geq D_2\\
| |
| \end{cases}
| |
| </math>
| |
| | |
| where:
| |
| | |
| :<math>\theta = \arctan d_{\rm AR}</math>
| |
| | |
| and <math>d_{\rm AR}</math> is the representative aspect ratio of the particle population, the ratio of the ''second longest'' to the ''longest'' dimensions of a particle (m/m)
| |
| | |
| The aspect ratio property, <math>d_{\rm AR}</math>, allows for the balanced screening of 'flaky' or 'elongated' particles on slotted meshes. Dehghani et al.'s relation reduces to Whiten and White's original equation when <math>\bar d_{\rm AR} = 1</math>.
| |
| | |
| Firth and Hart (2008) suggested a modification to the partition curve to account for the observed entrainment of fine particles in an oversize stream, with decreasing probability as particle size increases:{{Firth and Hart (2008)}}
| |
| | |
| :<math>E_{{\rm oa}i} = E_{{\rm oc}i} + \left ( 1 - E_{{\rm oc}i} \right ) \cdot R_{\rm f} \exp(-\lambda \bar d_i)</math>
| |
| | |
| where:
| |
| * <math>E_{{\rm oa}i}</math> is the actual fraction of particles of size interval <math>i</math> in the feed reporting to the oversize stream (frac)
| |
| * <math>R_{\rm f}</math> is the fraction of feed liquids split to the oversize stream (frac)
| |
| * <math>\lambda</math> is the size constant
| |
|
| |
|
| == Excel == | | == Excel == |
Revision as of 12:35, 28 April 2023
Description
This article describes the Whiten and White (1977) expression for the partition of particles to oversize during screening.[1][2] Several additions are made to the expression to reflect fines entrainment and particle shape.
The Whiten and White formulation is convenient for modelling screens as it implicitly honours the aperture size limit on particle passage, unlike other commonly used representations such as the Whiten efficiency curve or Rosin-Rammler equation.
Model theory
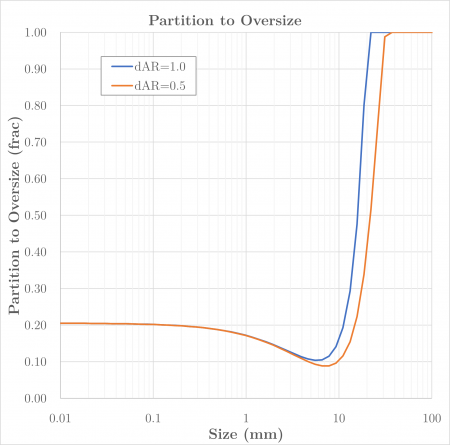
Figure 1. Screen partitions to oversize, with

,

,

and

. Note how the particle aspect ratio,

, shifts the partition curve, allowing irregular particles to pass slotted apertures that would otherwise be retained.
The Whiten and White expression for recovery to screen oversize is:
![{\displaystyle E_{{\rm {oc}}s}={\begin{cases}\exp(-n_{L}Lf_{\rm {o}}{\dfrac {{\big [}D_{1}-s{\big ]}{\big [}D_{2}-s{\big ]}}{D_{1}D_{2}}})&s<D_{2}\\1.0&s\geq D_{2}\\\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b064eb8f4289908faa6102b0d66e617f4de33c71)
where:
 is the diameter of a particle (mm)
is the diameter of a particle (mm) is the corrected fraction of particles of size
is the corrected fraction of particles of size  in the feed reporting to the oversize stream (frac)
in the feed reporting to the oversize stream (frac) is the number of trials per unit length parameter (/m)
is the number of trials per unit length parameter (/m) is the length of the screening area (or panel) in the direction of flow (m)
is the length of the screening area (or panel) in the direction of flow (m) is the fraction open area of the screen deck/panels (m2/m2)
is the fraction open area of the screen deck/panels (m2/m2) is length (the longer side) of the screen deck/panel aperture (m)
is length (the longer side) of the screen deck/panel aperture (m) is width (the shorter side) of the screen deck/panel aperture (m)
is width (the shorter side) of the screen deck/panel aperture (m)
Excel
The Whiten and White partition model may be invoked from the Excel formula bar with the following function call:
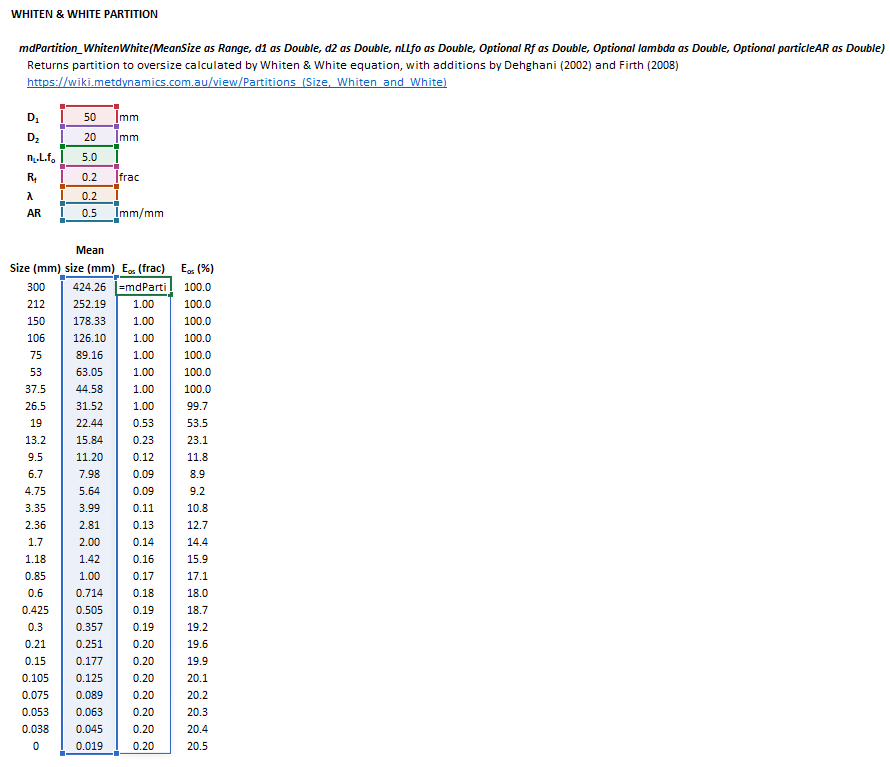
=mdPartition_WhitenWhite(MeanSize as Range, d1 as Double, d2 as Double, nLLfo as Double, Optional Rf as Double = 0, Optional lambda as Double = 0, Optional particleAR as Double = 1)
Invoking the function with no arguments will print Help text associated with the model, including a link to this page.
The input parameters and model results are defined below in matrix notation, along with an example image showing the selection of the same cells in the Excel interface:
SysCAD
 This section is currently under construction. Please check back later for updates and revisions. This section is currently under construction. Please check back later for updates and revisions.
|
References
- ↑ Whiten, W.J. and White, M.E., 1977. Modelling and simulation of high tonnage crushing plants. In: Proceedings of the 12th International Mineral Processing Congress, vol. II. Sao Paulo, pp. 148–158.
- ↑ Dehghani, A., Monhemius, A.J. and Gochin, R.J., 2002. Evaluating the Nakajima et al. model for rectangular-aperture screens. Minerals engineering, 15(12), pp.1089-1094.






![{\displaystyle E_{{\rm {oc}}s}={\begin{cases}\exp(-n_{L}Lf_{\rm {o}}{\dfrac {{\big [}D_{1}-s{\big ]}{\big [}D_{2}-s{\big ]}}{D_{1}D_{2}}})&s<D_{2}\\1.0&s\geq D_{2}\\\end{cases}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b064eb8f4289908faa6102b0d66e617f4de33c71)







![{\displaystyle {\begin{aligned}MeanSize&={\begin{bmatrix}{\bar {d}}_{1}{\text{ (mm)}}\\\vdots \\{\bar {d}}_{n}{\text{ (mm)}}\\\end{bmatrix}}\\\\d1&={\big [}D_{1}{\big ]}\\d2&={\big [}D_{2}{\big ]}\\nLLfo&={\big [}n_{\rm {L}}.L.f_{\rm {o}}{\big ]}\\Rf&={\big [}R_{\rm {f}}{\big ]}\\lambda&={\big [}\lambda {\big ]}\\particleAR&={\big [}d_{\rm {AR}}{\big ]}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cb9304fdc803b8545ca8b6ab31aa443c59f1072e)