Tumbling Mill (Power, Hogg and Fuerstenau): Difference between revisions
md>Scott.Munro m (→Excel) |
imported>Scott.Munro m (→Model theory) |
||
| (3 intermediate revisions by 2 users not shown) | |||
| Line 5: | Line 5: | ||
== Model theory == | == Model theory == | ||
{{Restricted content}} | |||
<hide> | |||
[[File:TumblingMillDimensions1.png|thumb|450px|Figure 1. Tumbling mill profile showing the charge shape and lift angle assumptions of the Hogg and Fuerstenau power draw model.]] | [[File:TumblingMillDimensions1.png|thumb|450px|Figure 1. Tumbling mill profile showing the charge shape and lift angle assumptions of the Hogg and Fuerstenau power draw model.]] | ||
| Line 28: | Line 31: | ||
\begin{cases} | \begin{cases} | ||
\dfrac{(1-f_{\rm v}) \rho_{\rm b}J_{\rm b} + (1-f_{\rm v}) \rho_{\rm m} (J - J_{\rm b}) + \rho_{\rm p} J_{\rm p} f_{\rm v} J}{J} & \text{for autogenous or semi-autogenous mills}\\ | \dfrac{(1-f_{\rm v}) \rho_{\rm b}J_{\rm b} + (1-f_{\rm v}) \rho_{\rm m} (J - J_{\rm b}) + \rho_{\rm p} J_{\rm p} f_{\rm v} J}{J} & \text{for autogenous or semi-autogenous mills}\\ | ||
\dfrac{(1-f_{\rm v}) \rho_{\rm b}J_{\rm b} + \rho_{\rm p}J_{\rm p}f_{\rm v}J_{\rm b} + \rho_{\rm p}(J-J_{\rm b})}{J} & \text{for ball mills} | \dfrac{(1-f_{\rm v}) \rho_{\rm b}J_{\rm b} + \rho_{\rm p}J_{\rm p}f_{\rm v}J_{\rm b} + \rho_{\rm p}(J-J_{\rm b})}{J} & \text{for ball or rod mills} | ||
\end{cases} | \end{cases} | ||
</math> | </math> | ||
| Line 79: | Line 82: | ||
Lift angle may alternatively be employed as a 'fitting parameter' for existing power draw measurements and subsequently used to predict power draw under differing conditions, at the user's discretion. | Lift angle may alternatively be employed as a 'fitting parameter' for existing power draw measurements and subsequently used to predict power draw under differing conditions, at the user's discretion. | ||
--> | --> | ||
</hide> | |||
== Excel == | == Excel == | ||
| Line 107: | Line 111: | ||
\rho_{\rm m}\text{ (t/m}^{\text{3}}\text{)}\\ | \rho_{\rm m}\text{ (t/m}^{\text{3}}\text{)}\\ | ||
\rho_{\rm b}\text{ (t/m}^{\text{3}}\text{)}\\ | \rho_{\rm b}\text{ (t/m}^{\text{3}}\text{)}\\ | ||
f_{\rm v}\text{ (v/v)}\\ | |||
\end{bmatrix},\;\;\;\;\;\; | \end{bmatrix},\;\;\;\;\;\; | ||
| Line 153: | Line 158: | ||
|- | |- | ||
|MillType | |MillType | ||
|style="background: #eaecf0" | AG/SAG or Ball | |style="background: #eaecf0" | AG/SAG or Ball/Rod | ||
|Type of mill, AG/SAG (''RockMass'', no ''AboveBallsSLMass'') or Ball (''AboveBallsSLMass'', no ''RockMass''). | |Type of mill, AG/SAG (''RockMass'', no ''AboveBallsSLMass'') or Ball (''AboveBallsSLMass'', no ''RockMass''). | ||
|- | |- | ||
| Line 199: | Line 204: | ||
|style="background: #eaecf0" | Input/Display | |style="background: #eaecf0" | Input/Display | ||
|Density of balls. | |Density of balls. | ||
|- | |||
|Voidage | |||
|style="background: #eaecf0" | Input/Display | |||
|Volumetric fraction of interstitial void space in the charge | |||
|- | |- | ||
|ChargeVolume | |ChargeVolume | ||
Revision as of 11:12, 4 December 2024
Description
This article describes the Hogg and Fuerstenau (1972) method for estimating the power draw of a tumbling mill.[1]
Model theory
Excel
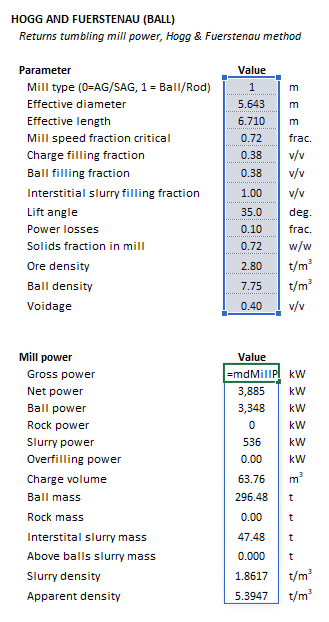
The Hogg and Fuerstenau mill power model may be invoked from the Excel formula bar with the following function call:
=mdMillPower_HoggFuerstenau(Parameters as Range)
Invoking the function with no arguments will print Help text associated with the model, including a link to this page.
The Parameters array and model results are defined below in matrix notation, along with an example image showing the selection of the same arrays in the Excel interface:
|
|
Please note the Excel function expects the mill diameter () and length () values in units of meters, which are internally converted to feet for use in the power equation presented above.
SysCAD
The Hogg and Fuerstenau power model is an optional calculation for tumbling mill units. If selected, the input and display parameters below are shown.
See also
References
- ↑ Hogg, R., 1972. Power relationships for tumbling mills. AIME Trans., 252, pp.418-423.